Trigonometry in chemistry
Tips for teaching maths skills to our future chemists, by Paul Yates of Keele University
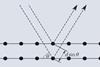
Given that chemistry takes place in three dimensions, it is of no surprise that trigonometry plays an important role in a mathematical description of the subject. Indeed, what may be most surprising is that only a very limited range of trigonometric skills is required by most chemists.
Measurement of angle
We normally measure angles in degrees, and this is the accepted convention when dealing with three dimensions in chemistry. However, there are other situations when a different angle unit is more convenient. This is the radian, which is related to the degree by the simple relationship

where π is the familiar constant which for most purposes can be taken as having the value of 3.142 or 22/7.
Thanks for using Education in Chemistry. You can view one Education in Chemistry article per month as a visitor.

Register for Teach Chemistry for free, unlimited access
Registration is open to all teachers and technicians at secondary schools, colleges and teacher training institutions in the UK and Ireland.
Get all this, plus much more:
- unlimited access to resources, core practical videos and Education in Chemistry articles
- teacher well-being toolkit, personal development resources and online assessments
- applications for funding to support your lessons
Already a Teach Chemistry member? Sign in now.
Not eligible for Teach Chemistry? Sign up for a personal account instead, or you can also access all our resources with Royal Society of Chemistry membership.


