Investigating activation energies
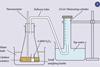
A challenge for post-16 students to investigate the activation energies of the enzyme-catalysed and the inorganic-catalysed decomposition of hydrogen peroxide
In our discussions with secondary school teachers in Hong Kong we are told that students, aged 16-17, generally find it difficult to plan their own experiments to determine activation energies for reactions. To address this problem we have devised a laboratory-based investigation which challenges students to compare the activation energies for the catalase-catalysed and iodide-catalysed decomposition of hydrogen peroxide. This investigation should also help them to understand the different effects of biological and inorganic catalysts on activation energy. To make the investigation more authentic, simulating the work of a biochemist for example, students extract catalase from a natural source, in this case lettuce leaves. Our trials demonstrated that lettuce produces a suitable concentration of catalase for this kind of investigation.
Thanks for using Education in Chemistry. You can view one Education in Chemistry article per month as a visitor.

Register for Teach Chemistry for free, unlimited access
Registration is open to all teachers and technicians at secondary schools, colleges and teacher training institutions in the UK and Ireland.
Get all this, plus much more:
- unlimited access to resources, core practical videos and Education in Chemistry articles
- teacher well-being toolkit, personal development resources and online assessments
- applications for funding to support your lessons
Already a Teach Chemistry member? Sign in now.
Not eligible for Teach Chemistry? Sign up for a personal account instead, or you can also access all our resources with Royal Society of Chemistry membership.


