Making ice cream - it's physical chemistry

Simply mixing cream, sugar and egg yolks, with a flavour such as fruit or chocolate, and putting them in the freezer won't give you ice cream. How these ingredients are processed will affect the texture of the product and this comes down to understanding the physical chemistry of ice cream.

Look at ice cream magnified several hundred times in a scanning electron microscope and you will see that it has a complex structure on length scales of 1 μm to 1 mm (Fig 1). There are ice crystals (ca 30 per cent by volume), air bubbles (50 per cent) and fat droplets (5 per cent) from the cream, held together by a viscous sugar solution (15 per cent). Ice cream thus contains all three states of matter simultaneously and is both a foam and an oil-in-water emulsion. The quality of ice cream depends on its microstructure: small ice crystals and air bubbles give the ice cream a smooth, soft texture. If the ice crystals are too large, the ice cream becomes gritty and unpleasant to eat. Creating this microstructure is the key to making good ice cream - and to understand how to do this, you need to know some physical chemistry.

Creating a microstructure

The first step is to know how much of each ingredient to use. lce cream typically contains ca 60 per cent ice by weight. Much more than this and the product would be too hard; much less it would be like custard and would not cool you down on a hot day. The amount of sugar (sucrose, C₁₂H₂₂O₁₁) determines the amount of ice in the ice cream because this will affect the freezing point.

Enter François-Marie Raoult

Pure water freezes at 0°C, but when a solute (eg sugar) is added, the freezing point of the solution is lowered ('depressed'), because the presence of solute molecules makes it harder for water molecules to order onto a crystal lattice, ie to freeze. (Salt is put on roads in winter to remove ice.) Our understanding of solution phenomena such as freezing point depression owes much to François-Marie Raoult (1830-1901), who measured the freezing points and other physical properties of many solutions. He showed that there was a linear relationship between the freezing point and the alcohol content of drinks. The freezing point depression (ΔT) depends on the concentration of solute molecules, but not their type, and is given by:

ΔT = T_w- T_s = (RT_w²/ ΔH_f) x

where T_w is the freezing point of pure water (273 K), T_s is the freezing point of the solution, R is the gas constant, ΔH_f  is the latent heat of fusion of water (6.01 kJ mol^-I) and x  is the mole fraction of solute. (The related observation that the vapour pressure of a solvent above a solution is proportional to the mole fraction of the solvent in solution is Raoult's Law.) Most solutions obey this equation when the solute concentration is low, but may diverge from it at high concentrations.

Making use of the freezing point curve 

Figure 2 shows the freezing point of sucrose solutions as a function of concentration. The freezing point depression deviates from linearity above mole fractions of ca 0.02. If you cool a sucrose solution below its freezing point, ice forms and removes water from the solution; the sucrose concentration therefore increases and the freezing point decreases further. As the temperature is reduced, more ice forms, and the solution concentration follows the curve. At any temperature below the freezing point, there is a given amount of ice in equilibrium with the freeze-concentrated sucrose solution.

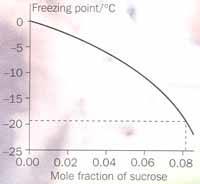
Ice cream scientists use the freezing point curve when formulating ice cream recipes. Suppose, for example, they want to make 1 kg of ice cream that contains 50 per cent ice by weight at a normal freezer temperature of -18°C. How much sucrose should they use? From the curve, the sucrose mole fraction in equilibrium with ice at -18°C is 0.083 or 63 per cent w/w, ie:

M_sucrose/(M_sucrose + M_water) = 0.63

The total mass is 1 kg, and 50 per cent of this will be ice, so the remainder, must be sucrose and unfrozen water.

M_sucrose + M_water = 500 g

Solving these two equations gives M_sucrose = 320 g. In practice, they also have to allow for other solutes, eg  from milk, when formulating ice cream recipes.

At the factory, the ingredients are first blended in the correct quantities and this mix is pasteurised to kill any harmful microorganisms. Initially, the fat is present as coarse globules. The mix is homogenised by forcing it through a small hole under high pressure (Fig 3 (a)).  This breaks the fat globules up into an emulsion of much smaller droplets (<1μm) with a much larger surface area. To keep the fat droplets small the emulsion must be stabilised with a surfactant (see Box 1). There are two types of surfactant in ice cream: milk proteins (eg casein) and emulsifiers (eg  mono-diglycerides or lecithin from egg yolks or soy beans). These adsorb to the surface of the homogenised fat droplets and stabilise the emulsion (Fig 3 (b)). The mix is then cooled to ca 5°C, below the melting point of the fat, which begins to crystallise.

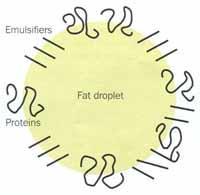
Thus one component of the microstructure - the fat droplets - has been created. In the next stage the mix is converted into ice cream by aerating and freezing it.

The Mongolian horsemen

Legend has it that making ice cream by simultaneously freezing and aerating cream originated when Mongolian horsemen took animal intestines filled with cream to eat on journeys across the Gobi desert in winter. As they galloped the cream was vigorously shaken, while at the same time the sub-zero temperatures froze it, creating ice cream.

Modern ice cream freezers are less exotic. They consist of a refrigerated barrel, with a rotating dasher inside, which is equipped with scraper blades. Ice cream mix at ca 4°C is pumped into the barrel. Air is injected, forming large bubbles, which are broken down into many smaller ones by the beating of the dasher. The fat droplets and milk proteins adsorb onto the surface of the air bubbles and stabilise them, in the same way that the milk protein and emulsifiers stabilise the fat droplets. Since the fat droplets are partially crystalline, they form a strong, rigid coating, which prevents collapse of the air bubbles.

The barrel wall is cooled to ca -30°C, so that when the mix touches it, ice forms instantly, and heat is rapidly extracted from the ice cream mix (see Box 2). The ice is rapidly scraped off the barrel wall by the rotating scraper blades, and is dispersed into the mix as small crystals. As the ice cream passes through the freezer, its temperature drops, more ice is formed, and the viscosity of the ice cream increases. There are two reasons for this increase in viscosity.

• First, the intrinsic viscosity of the sugar solution decreases as it gets colder. 
• Secondly, as Einstein originally pointed out, the viscosity of a suspension of solid particles increases as the volume fraction of solid (ie ice) increases. This means that the mix gets harder to beat, and the energy input needed to rotate the dasher is greater. This energy is dissipated in the ice cream as heat. Eventually, when the temperature of the ice cream reaches ca  -5°C, the energy input through the dasher equals the energy removed as heat by the refrigerant, and it is not possible to cool the ice cream any further: the process becomes self-limiting. At -5°C, the ice cream is too soft to process, by covering it in chocolate for example, and the microstructure is unstable. Therefore the ice cream is extruded from the freezer and cooled rapidly ('hardened') by blowing air at ca  -40°C over it in an enclosed chamber. The size of the ice crystals (and thus the quality of the final product) depends on the conditions inside the freezer, such as the wall temperature, and the amount of time the ice cream spends in the barrel, and the speed at which it is hardened.            

The journey home

After hardening, the ice cream is packaged and transported from the factory to the shop. It is important that the microstructure of the ice cream is preserved during its journey. Ice cream is temperature sensitive: if it warms up too much, it melts. However, even at temperatures below its melting point, the quality can deteriorate, owing to changes in the microstructure (see Fig 4).

We have already seen that dispersions of one phase in another are unstable and have a tendency to lower their energy by forming fewer, larger particles. In emulsions this usually occurs by the coalescence of two drops. However, when the dispersed phase is soluble in the continuous phase, another mecha?nism operates. This is known as Ostwald ripening, discovered by Wilhelm Ostwald, who won the 1909 Nobel Prize in chemistry for this work. The solubility of a particle in?creases as the particle gets smaller, ie  small particles are more likely to dissolve than large ones. The solubility of a particle of radius r, s(r)  is given by:

In[s (r)/s (∞)] = [2γ V/RT] [1/r]

where V  is the molar volume and s (∞) is the solubility of an infinitely large particle. This is known as the Gibbs-Thomson equation. (William Thomson, later Lord Kelvin, is best known for proposing the absolute scale of temperature.)

When you take ice cream out of a supermarket freezer its temperature may rise by a few degrees during your journey home, so that some of the ice melts. Crystals that are initially large become smaller, and those that are initially small disappear completely. When you put the ice cream in your freezer at home, it is cooled down again, and the ice that had melted freezes again (Fig  4). However, the ice cannot form on the crystals that have disap?peared. Instead it freezes on the crystals that have survived. The net effect is that the total number of crystals is reduced, and their mean size increases, while the total amount of ice is unchanged. If the temperature fluctuation is too big, the ice crystals become very large (> ~100 μm),  producing an icy, gritty texture.

So having created and preserved the microstructure, the ice cream finally reaches the consumer in perfect condition. The next time you eat ice cream, think of Raoult, Gibbs, Newton, Kelvin and Ostwald - and all the physical chemistry that has gone into making it!

Dr Chris Clarke  is a research scientist at Unilever R  & D Colworth, Sharnbrook, Bed?ford MK44 lLQ.

Also of interest