Symmetry of buckminsterfullerene
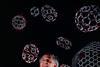
Both Euler's formula and Descartes' theorem can be used to show how buckyballs are made from closed cages of carbon pentagons and hexagons
Buckminsterfullerene, C60, is one member of the family of fullerenes, pure carbon molecules that are a cage of carbon atoms. These cages can be closed (buckyballs, fig 1) or open (buckytubes, fig 2). They were first reported in 19851 and were the subject of the Nobel prize for chemistry in 1996.2 In the original experiment when graphite underwent laser ablation, C60 was by far the most prevalent member of the fullerenes, with C70 being the second most abundant.2 C60 contains 12 pentagons and 20 hexagons, fused such that the centres of the pentagons are at the corners of an icosahedron, making buckminsterfullerene a truncated icosahedron. Each of the pentagons shares its edges with adjacent hexagons.
Thanks for using Education in Chemistry. You can view one Education in Chemistry article per month as a visitor.

Register for Teach Chemistry for free, unlimited access
Registration is open to all teachers and technicians at secondary schools, colleges and teacher training institutions in the UK and Ireland.
Get all this, plus much more:
- unlimited access to resources, core practical videos and Education in Chemistry articles
- teacher well-being toolkit, personal development resources and online assessments
- applications for funding to support your lessons
Already a Teach Chemistry member? Sign in now.
Not eligible for Teach Chemistry? Sign up for a personal account instead, or you can also access all our resources with Royal Society of Chemistry membership.


