The Power of NMR: The Beginnings

By 2008-07-01T00:00:00

image - feature - brown fig 1

Originally a curiosity of the quantum world, NMR is now an essential tool for chemists, biochemists and clinicians

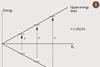
Nuclear magnetic resonance (NMR) spectroscopy involves the excitation of nuclei by electromagnetic radiation in the radio-frequency range of the electromagnetic spectrum. For a nucleus to absorb energy from radiowaves in this way, it must have the quantum mechanical property of spin. A spinning nucleus, such as that of the hydrogen atom, will adopt one of only two possible states when placed in a magnetic field. (In NMR, the hydrogen nucleus is often referred to as a proton, and is given the abbreviation 1 H.) As the strength of the magnetic field is increased, there is a proportional increase in the energy 'gap' between these two states, as is shown in Fig 1. We can predict the resonant frequency at which any spinning nucleus will absorb energy from radio-frequency radiation (ν), as it jumps from the lower energy state to the upper state, by using the fundamental equation: 

ν = γB0/2π       (i)

where  B0 is the magnetic field which the nucleus experiences, and ν is a proportionality constant, which is specific to that nucleus.

Thanks for using Education in Chemistry. You can view one Education in Chemistry article per month as a visitor. 

A photograph of a teacher standing in a white lab coat, speaking with a class of children in a laboratory, is superimposed on a colourful background. Text reads "Teach Chemistry means support for classroom and staff room".

Register for Teach Chemistry for free, unlimited access

Registration is open to all teachers and technicians at secondary schools, colleges and teacher training institutions in the UK and Ireland.

Get all this, plus much more:

  • unlimited access to resources, core practical videos and Education in Chemistry articles
  • teacher well-being toolkit, personal development resources and online assessments
  • applications for funding to support your lessons

Set up your Teach Chemistry account

Already a Teach Chemistry member? Sign in now.

Not eligible for Teach Chemistry? Sign up for a personal account instead, or you can also access all our resources with Royal Society of Chemistry membership.