Mike Follows shows how relativity has affected gold and mercury, and asks if it will affect elements yet to be discovered
Most scientists may be surprised to learn that Einstein’s theory of special relativity causes some elements to behave out of character, breaking the periodic law of the chemical elements. For example, gold owes its beautiful colour to relativity, and mercury is a liquid at room temperature because of it. For one and a half centuries, the periodic table has doubled as a powerful tool and the most eye-catching emblem for science. But is relativity causing cracks to appear in Dmitri Mendeleev’s creation, and are these cracks likely to widen as more elements are synthesised?

Several chemists recognised periodicity in the behaviour of the elements, but it was Mendeleev who took the accolade in 1869. His table included the 63 elements known at the time but, in order to improve the observed periodicity, he swapped some elements, including tellurium with iodine, and presciently ranked them in order of increasing atomic number. He left several gaps. Not only were the gaps filled by elements such as gallium, germanium and scandium as implicitly predicted but also their properties closely matched what was expected. When Yuri Oganessian and his collaborators at the Joint Institute for Nuclear Research near Moscow announced they had synthesised the elusive element 117 (ununseptium) in 2010, their countryman’s table was now complete down to period 7.
Quantum mechanics 101
Atomic structure is usually taught as a historical progression and we learn to be wary of treating orbiting electrons as particles; much safer to think of them as waves, which is why the Rutherford model was quickly abandoned. After all, a charged particle orbiting the nucleus must continuously change direction. According to Newton’s first law of motion, this means it must be accelerating. Conventional electrodynamic theory dictates it must radiate electromagnetic energy and crash into the nucleus, leading to the collapse of the atom within 10-10 s.
This ushered in the Bohr model. Published in 1913, it appeared to side-step this problem by prohibiting all orbits except those with quantised angular momentum (see Box 1). In 1924, Louis de Broglie applied wave-particle duality to electrons and represented them as standing waves. The different energy levels of the electrons corresponded to different harmonics of these waves, like notes plucked on a guitar string. The idea of electrons as waves appeared to be cemented when the Austrian Erwin Schrödinger developed his wave equation in 1926, which led to the strangely-shaped orbitals reminiscent of twisted balloons. But, in the meantime, the German Werner Heisenberg had developed a model based on matrix mechanics that treated electrons as particles, though his uncertainty principle suggested electrons could be imagined as probability clouds spread throughout Schrödinger’s orbitals.
Then in 1928, the British physicist Paul Dirac developed a third complete quantum theory and showed those of Schrödinger and Heisenberg were mathematically equivalent to each other and were contained within his model. In other words, electrons appeared to display wave-particle duality but behaved as particles and waves at the same time. This suggested that the Bohr model of the atom, a rather ad hoc synthesis of classical and quantum physics, was closer to reality than is generally recognised and it had the merit of providing the basis for understanding chemistry. Electronic configuration was born out of the Bohr model (see Box 2).
Why is mercury a liquid?
Can the behaviour of normal metals shed any light on the behaviour of mercury? A normal metal can be visualised as a lattice of positive ions ‘glued together’ by a ‘sea’ of shared valence electrons. These delocalised electrons explain why metals are good conductors – of both heat and electricity. Metals are ductile and malleable because these electrons bind the ions even when they are sliding past each other. The more valence electrons donated to the ‘sea’, the stronger this glue – magnesium donates two electrons per atom so is harder and has a higher melting point than sodium, which donates only one electron.
Mercury represents an extreme case and is liquid at room temperature. Part of the reason is its electronic configuration. The two electrons in its 6s orbital are happily paired up with each other and are reluctant to be shared among neighbouring atoms. This causes mercury to behave like a noble gas. It is the only metal that is monatomic in the gas phase, not forming diatomic molecules like its cousins.
Mercury is reluctant to oxidise and is a poor conductor compared to other members of its group. But cadmium, sometimes known as ‘non-relativistic’ mercury because it is directly above it in the periodic table, has a melting point of 321°C, so why does mercury have a melting point of –39°C? Relativistic contraction of the 6s orbital provides the coup de grâce.
How does relativity creep in?
Dirac showed that all electrons have angular momentum, providing implicit endorsement of the Bohr model. From the Bohr model, we know the velocity of an electron is proportional to the atomic number, Z, of an element (see Box 1) and the speed of an orbiting electron can be expressed approximately as:

where c is the speed of light.
In his 1905 paper on relativity, Einstein postulated that the mass of a moving object (m) increases with its velocity (v) according to the equation:
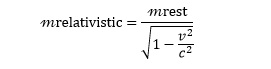
The fact that particles ‘put on weight’ as they move faster explains why they cannot break the speed of light – at this speed, they would have infinite mass and would then require infinite energy to accelerate them.
Using the equations above for mercury, the electron orbits at 58% the speed of light and, as a consequence of relativity, its mass increases by 23%. This causes its orbital radius to contract by 23% and results in a stronger attraction between the nucleus and the electrons.
Indeed, in 2013, an international team led by Peter Schwerdtfeger used quantum molecular dynamics to calculate the heat capacity of mercury. When they included relativistic effects, their calculation for the melting point closely matched the observed value of –39°C compared to 82°C (or 355K) without relativity.
The colour of gold
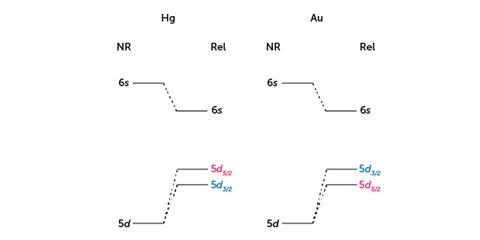
It was only as recently as the 1960s that Pekka Pyykkö discovered that relativity accounts for the colour of gold. We are taught in school that each group has a typical chemical behaviour that can be traced to the valence electron. Much later, we learn that similarities between elements along each period can be just as important. The d-block transition metals, for example, are typically metallic in colour. Indeed, across the sixth period, the relativistic contraction of the 6s orbital increases and reaches a sharp maximum at gold, known as the ‘group 11 maximum’. So given that relativity plays a role in the behaviour of both mercury and gold, why do they have such different properties when they are ‘next-door neighbours’ on the periodic table?
The answer lies in the difference in their electronic configurations. Gold has a half-filled 6s orbital. When a typical d-block metal absorbs a UV photon, an electron is excited from a d to an s orbital. Photons of visible light are reflected because they do not have enough energy to promote an electron in this way. This is why metals such as silver look and act like mirrors. Relativity causes the s and p orbitals to contract, which screens the d and f orbitals from the nucleus, so they experience a smaller electrostatic force and their orbits get bigger. This lowers the energy of the 6s orbital and raises the 5d orbital, narrowing the gap between the two levels. This means gold atoms can be excited by blue photons, while other colours bounce off the surface, so we observe white light minus blue – which gives us the characteristic golden-yellow hue.
More consequences of relativity
In the lower third of the periodic table, the chemical characteristics of the elements are strongly influenced by relativity. For example, lighter group 13 and 14 elements such as Al and Ge obey normal valency rules that favour oxidation states of III and IV, respectively. Relativistic contraction in the heavier members of group 13 and 14 (such as Tl and Pb) means the two electrons in the 6s orbital are essentially an ‘inert pair’ and reluctant to participate in bonding, so oxidation states I and II are favoured.
A team of physicists and chemists that included Pyykkö claimed that ‘cars start due to relativity’ because relativity accounts for 85% of the voltage in a 2 V lead–acid battery. Relativity has a smaller effect on tin (just above Pb in the periodic table) and this explains why tin batteries were never developed.
What does the future hold?
Elements beyond atomic number 103 need to be synthesised in particle accelerators where heavy nuclei are fused together. The discovery of element 117 was based on the observation of just six atoms, though a total of 15 have been created to date. Because these synthesised atoms are very short-lived, traditional ‘wet’ chemistry is impossible. This means that researchers need to conjure up ingenious ways to infer the chemistry of these transient elements.

According to the periodic law of the elements, rutherfordium (element 104) and dubnium (105) should behave like the elements directly above them in the periodic table – hafnium (72) and tantalum (73). Instead, Ken Czerwinski found rutherfordium has more in common with plutonium (94) while dubnium is like protactinium (91), which are remote from each other on the table. However, seaborgium (106) and bohrium (107) behave exactly as Mendeleev would have predicted.
Relativistic contraction occurs along period 7 as it does in period 6, but the maximum occurs at eka-mercury and not below gold as expected. It remains to be seen whether element 112 exhibits the effects of relativity. So perhaps the jury is still out on whether relativity will undermine Mendeleev’s periodicity as new elements are added. Regardless, the immediate future looks exciting.
Elements 119 and 120 would fill the s orbital of the new eighth period. Perhaps even more significantly, element 121 would start the g orbitals never filled before, a new block that will accommodate 18 electrons and broaden the table to 50 columns.
What is the maximum number of elements? Richard Feynman suggested that element 137 might be the limit because bigger atoms would require 1s electrons to exceed light-speed. Scientists who have taken account of the volume of the nucleus estimate the final element to have an atomic number of 172 or 173.
Given the lifetime of artificial atoms is so fleeting, perhaps the enterprise of synthesising new elements is little more than academic curiosity. Whether or not relativity interferes with the expected periodicity of new elements does not undermine Mendeleev’s table in any practical sense. So chemists can continue displaying the periodic table with pride in the knowledge the atomic world is even more fascinating, if only because it has a slice of relativity. When you next admire some gold jewellery, marvel at the fact that its atoms are like nanoscopic perpetual motion machines with electrons whizzing around at 58% the speed of light. And every time you turn the key in the ignition for the morning commute, remember you are harnessing the relativity that lurks inside atoms of lead.
Mike Follows is a teacher at King Edward’s School in Birmingham, UK
Box 1 – The mathematics of the Bohr model
The maths from the Bohr model is a marriage of classical and quantum mechanics.
From classical mechanics, electrostatic attraction (the coulomb force) provides the centripetal force for the orbiting electron:
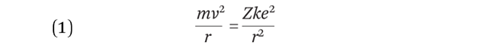
where m is the mass of the electron, e is the electronic charge, k is the coulomb constant and Z is the atomic number. This can be rearranged for the velocity of the electron:
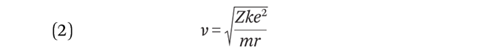
The total energy of the electron is the sum of its kinetic and potential energies.
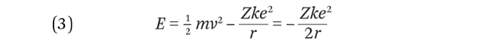
But Bohr introduced quantum physics by postulating that the angular momentum of the electron is quantised as:
![]()
Substituting (2) into (4) gives an equation for orbital radius in terms of n:
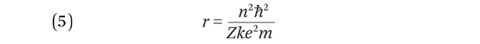
The smallest orbital radius in the hydrogen atom is called the Bohr radius and is 5.3 x 10–11 m. Expressing (4) in terms of v and then substituting for r using equation (5) gives:

So the velocity is proportional to the atomic number. By substituting values for the constants, this equation reduces to:
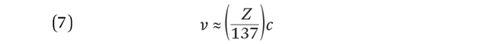
Box 2 – Electronic configuration
Successive electron shells should contain 2, 8, 18, or 2n2 electrons in general, where n denotes the shell number and the factor of 2 is a consequence of the Pauli exclusion principle. Each shell includes one or more subshells, labelled s, p, d, f and g, corresponding to values l = 0, 1, 2, 3 etc, and the maximum number of electrons that can be placed in a subshell is given by 2(2l + 1).
Electrons fill the energy levels in order of increasing energy levels. Based on the Bohr model, the aufbau principle (from the German to ‘build up’) or diagonal rule was first stated by Charles Janet in 1929. Every two periods, a new family of electron orbitals appears. Compared to the conventional Mendeleev table, Janet’s left-step periodic table is tied more closely to quantum mechanics and the order of electron shell filling. For atoms more massive than hydrogen, the situation is complicated by the interaction between electrons so there is no way of knowing the lowest energy configurations a priori but it works perfectly in about 80% of cases. It gives the following order for filling the sub-shells:
Pekka Pyykkö used computer modelling to work out the electronic configuration all the way up to Z = 172 and suggests that sub-shells in period 8 will be filled in the order 8s, 5g, first 2 spaces of 8p, 6f, 7d, 9s, first 2 spaces of 9p and so on, which deviates from the diagonal rule.
| Electron shell, n | Sub-shells according to aufbau | Number of electrons |
|---|---|---|
| 1 | 1s | 2 |
| 2 | 2s, 2p | 8 |
| 3 | 3s, 3p | 8 |
| 4 | 4s, 3d, 4p | 18 |
| 5 | 5s, 4d, 5p | 18 |
| 6 | 6s, 4f, 5d, 6p | 32 |
| 7 | 7s, 5f, 6d, 7p | 32 |
| 8 | 8s, 5g, 6f, 7d, 8p, 9s | 50 |
Pekka Pyykkö used computer modelling to work out the electronic configuration all the way up to Z = 172 and suggests that sub-shells in period 8 will be filled in the order 8s, 5g, first 2 spaces of 8p, 6f, 7d, 9s, first 2 spaces of 9p and so on, which deviates from the diagonal rule.
1/2/2016 This article was updated to clarify that oxidation states I and II in theheavier members of group 13 and 14 are favoured due to relativistic contraction
References
- R Ahuja et al, Phys. Rev. Lett., 2011, 106, 018301 (DOI: 10.1103/PhysRevLett.106.018301)
- F Calvo et al, Angew. Chem. Int. Ed., 2013, 52, 7583 (DOI: 10.1002/anie.201302742)
- M Barysz and Y Ishikawa, Relativistic methods for chemists. Springer, 2010
- L J Norrby, J. Chem. Educ., 1991, 68, 110 (DOI: 10.1021/ed068p110)
- Scientific American, June 2013, p68








1 Reader's comment