Try this worked example of a Chemistry Olympiad question to practise writing equations and mole calculations in the context of explosives
In this question from the 2011 UK Chemistry Olympiad round one paper, students work with the general formula for certain high explosives, calculating the oxygen balance of RDX and writing the equation for the explosion of TNT. The resource features a video walkthrough to help students if they get stuck or want to check their working.
The question is of low difficulty, and covers the following chemistry topics:
- Quantitative chemistry
- Writing equations
- Mole calculations
What’s on this page?
- How to use this resource
- Question
- Answers
- Walkthrough (including video and transcript)
How to use this resource
Find out more about how to use Chemistry Olympiad worked answers.
Note
This question first appeared as question 3 in the 2011 UK Chemistry Olympiad round one paper. For marking, including notes on allowances, see question 3 in the 2011 examiners’ mark scheme.
Question
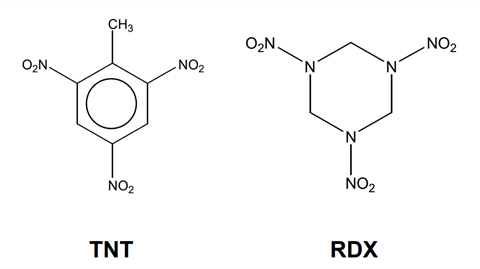
Explosives are often associated with causing destruction and harm, but explosives have also played a major role in civil engineering for hundreds of years. ‘Blasters’ (explosives engineers) need a good understanding of geology and the science of explosives to be able to design explosions ‘to order’.
Explosions occur when reactions proceed so that heat is generated more rapidly than it can be dispersed.
Some high explosives have the general formula Ca Hb Nc Od. The oxidiser (O) and the fuel (C, H) are present in the same molecule. Nitrogen atoms are present so that there is the highly exothermic production of hot N2 gas.
Part a
Find an expression for d (in terms of a, b and c) that must be satisfied for an explosive Ca Hb Nc Od to decompose to form CO2, H2 O and N2 only.
Part b
The oxygen balance of an explosive is the mass of oxygen either in excess (ie the balance is positive) or in deficit (ie the balance is negative) of that required for complete oxidation of C and H.
The oxygen balance is expressed as a percentage of the molar mass of the explosive.
Write a general expression for the oxygen balance of Ca Hb Nc Od.
Part c
Calculate the oxygen balance for RDX, whose structure is given above.
Part d
When the explosive does not contain enough oxygen, there is incomplete oxidation of C and/or H. In the case of nitrated aromatic compounds, the reaction products are given by the following set of ‘rules’:
- Carbon atoms react with the available oxygen to form CO
- N atoms combine to form N2
- 1/3 of the CO produced is converted to C + CO2
- 1/6 of the original CO produced reacts with any available H in the compound to form C + H2 O
Write the equation for the explosion of TNT.
Part e
Calculate the mass of TNT that will explode to produce 1.0 dm3 of gas at room temperature and pressure. (The molar volume of any gas = 24 dm3 at RTP.)
Answers
Part a
(Ca Hb Nc Od→ a CO2 + b/2 H2 O + c/2 N2)
d = 2a + b/2
Part b
O balance = 16 × (d – 2a – b/2) x 100%
(12a + b + 14c + 16d)
Part c
O balance = – [100 × (3 × 16) / 222.14] = –21.6%
Part d
C7 H5 N3 O6→ 3CO + 3C + CO2 + 3/2 H2 + 3/2 N2 + H2 O
Part e
Amount of gas = 1/24 mol
Molar ratio TNT: gas = 1:7
Amount of TNT = 1/7 × 1/24 mol = 1/168 mol = 0.0060 mol
Mass of TNT = 227.14 g mol–1× 1/168 mol = 1.35 g
Walkthrough
Transcript
Download this
Introduction
This question is all about explosives and how explosives work.
The question starts with an introduction into explosives and shows us the skeletal formulae of two common explosives, TNT and RDX. It states that some high explosives have a general formula of Ca Hb Nc Od. The oxidiser (the oxygen atoms) and the fuel (the carbon and hydrogen atoms) are all present in the same molecule. The nitrogen atoms are present so that there is the highly exothermic production of hot nitrogen gas.
Part a
Part (a) of the question asks you to find an expression for d in terms of a, b and c that must be satisfied for an explosive of general formula Ca Hb Nc Od to decompose to form CO2, H2 O and N2 only. To answer this question, we can begin by writing out a simple equation to represent the reaction taking place. The key information is that the oxidiser and the fuel are all present in the same molecule and hence the only reactant is the explosive itself. The products are CO2, H2 O and N2.
If we now balance the equation, we must produce a molecules of CO2, b/2 molecules of H2 O (as each water molecule has two hydrogen atoms) and c/2 molecules of N2. Now balancing the oxygen atoms, we have d atoms of oxygen on the left and 2a + b/2 atoms of oxygen on the right. Thus for an explosive of general formula Ca Hb Nc Od to decompose to form CO2, H2 O and N2 only, d = 2a + b/2.
Part b
The question now moves on to explain the meaning of the oxygen balance of an explosive and how it can be calculated. The oxygen balance of an explosive is the mass of oxygen either in excess or in deficit of that required for the complete oxidation of the carbon and hydrogen atoms present in the explosive, ie how much oxygen is present in the molecule compared to the amount needed for the complete combustion of all the fuel in the molecule. It is expressed as a percentage of the molar mass of the explosive.
Part (b) asks you to write a general expression for the oxygen balance of Ca Hb Nc Od. Remembering that the mass of a substance in grams is equal to the number of moles of that substance multiplied by its molar mass in g per mole, then the mass of oxygen present in the molecule Ca Hb Nc Od is equal to the number of moles of oxygen present, d, multiplied by the molar mass of oxygen which is 16 g per mole. This is the mass of oxygen present. The mass of oxygen required for the complete combustion of the explosive is effectively the mass of oxygen on the right hand side of the equation we wrote in part (a), since these are the products if complete combustion occurred. Thus the mass of oxygen required for the complete combustion of the fuel is the number of moles of oxygen produced = 2a + b/2 multiplied by the molar mass of oxygen, ie 16 g per mole. The difference between these two values is therefore 16d – 16(2a + b/2).
The question tells us that the oxygen balance is expressed as a percentage of the molar mass of the explosive. Again using the equation mass = moles times molar mass, the molar mass of the explosive can be calculated as 12a + b + 14c + 16d. Therefore the oxygen balance of Ca Hb Nc Od is 16d – 16(2a + b/2) all divided by 12a + b + 14c + 16d multiplied by 100%.
Part c
In part (c) of the question, you are asked to calculate the oxygen balance for RDX, whose structure is given at the start of the question. This can be calculated using the answer to part (b). In order to use this equation it is necessary to determine the molecular formula of RDX from its skeletal formula. If you have not come across skeletal formulae in your studies, at each junction between the bonds and at the end of each bond it is assumed that there is a carbon atom. In addition, the hydrogen atoms are not drawn in. It is therefore important that when determining a molecular formula from a skeletal formula that you add in the correct number of hydrogen atoms such that each atom has the correct number of bonds, ie each carbon atom must have four bonds.
It is best to draw out the structural formula of the molecule and then count up the number of carbon, hydrogen, nitrogen and oxygen atoms. If we do this, we determine that the molecular formula for RDX is C3 H6 N6 O6 and hence for substitution into our equation for oxygen balance, a = 3, b = 6, c = 6, d = 6.
To complete the question, it is now simply a matter of substituting each of these values into the equation. Thus, the oxygen balance of RDX is –21.6%.
Part d
The final part of the question describes what happens if an explosive does not contain enough oxygen. If this is the case, there is incomplete oxidation of C and/or H. For nitrated aromatic compounds the products are determined by a following a set of rules.
Part (d) of the question asks us to apply these rules to determine the products from the explosion of TNT and hence write an equation for its explosion. Once again, our first step is to determine the molecular formula of TNT from its skeletal formula. Drawing out the structural formula of TNT we can see that it contains 7 carbon atoms, 5 hydrogen atoms, 3 nitrogen atoms and 6 oxygen atoms and hence has a molecular formula of C7 H5 N3 O6. Before proceeding we can check that it does not contain sufficient oxygen for complete combustion by looking back at our answer to part (a). Here we determined that for complete combustion, the number of atoms of oxygen in the explosive must be equal to the two times the number of atoms of carbon plus half the number of atoms of hydrogen. In this case by doing a quick sum we can see that the oxygen content is insufficient and so incomplete combustion will occur. As TNT is a nitrated aromatic compound we can follow the rules to determine the products from its explosion.
Let’s follow each of the bullet points in turn. The first bullet point tells us that the carbon atoms react with the available oxygen to form CO. Thus, the 7 carbon atoms in TNT react with the six available oxygen atoms to form 6 molecules of CO and a single C atom. Following the second bullet point, the 3 nitrogen atoms combine to form 1½ molecules of N2. Looking now at the third and fourth bullet points, one third of the 6 molecules of CO formed (ie 2) are converted into C and CO2. In addition, one sixth of the 6 molecules of CO formed (ie 1) react with 2 atoms of hydrogen in TNT to form C and H2 O. Thus we can write the equation as C7 H5 N3 O6 forms 3CO + C + 1½ N2, + C + CO2, + C + H2 O. However finally we must balance the hydrogen atoms to form H2 gas. If we do this we determine the final equation for the explosion of TNT as:
C7 H5 N3 O6→ 3CO + 3C + 1½ N2 + CO2 + H2 O + 1½ H2
Part e
The last part of the question, part (e), asks us to calculate the mass of TNT that will explode to produce 1.0 dm3 of gas at room temperature and pressure. In order to do this, we need to use the equation for the explosion of TNT produced in part (d). At room temperature and pressure, of the products from the explosion, carbon monoxide, nitrogen, carbon dioxide and hydrogen will all be gases. Thus one mole of TNT explodes to produce seven moles of gas.
To use this fact, we now need to find out the number of moles in 1.0 dm3 of gas at RTP. If we know that the molar volume of any gas at RTP is 24 dm3, then we can calculate that the number of moles in 1.0 dm3 of gas is 1 dm3÷ 24 dm3 mol–1 = 0.0417 mol. Since one mole of TNT produces 7 moles of gas, then the number of moles of TNT which must have exploded to produce 0.0417 moles of gas is equal to 0.0417 moles divided by 7 = 5.95 × 10–3 moles of TNT.
The question however asks us to calculate the mass of TNT that will explode to produce this volume of gas. The molar mass of TNT can be calculated to be 227.14 g mol-1. Therefore, using the equation, mass = moles × molar mass, we can calculate the mass of 5.95 × 10-3 moles of TNT as 1.35 g. As we have only been given the accuracy of the volume of gas to 2 significant figures, our final answer should only be given to the same degree of accuracy. Therefore, the mass of TNT that will explode to produce 1.0 dm3 of gas at room temperature and pressure is 1.4 g.
Downloads
Writing ‘explosive’ equations - video transcript
Editable handout | Word, Size 0.98 mbWriting ‘explosive’ equations - video transcript
Handout | PDF, Size 0.66 mb
Chemistry Olympiad worked answers
- 1
- 2
- 3
 Currently reading
Currently readingWriting ‘explosive’ equations
- 4
- 5
- 6
- 7
- 8
























No comments yet